SIMTEACH
SIMTEACH
A design of these simulations has been sketched in the article by Chieu, V. M. and Herbst, P. (2011). Designing an intelligent teaching simulator for learning to teach by practicing. ZDM—The International Journal of Mathematics Education, 43(1), 105–117.
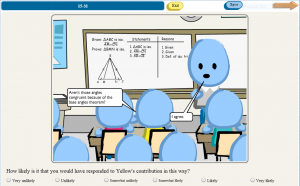
A major concern in the preparation of teachers is bridging course-based learning with practice in real classrooms. However, regular classrooms do not provide controlled situations where beginning teachers can focus on developing specific, important aspects of their teaching practice. The goal of this project was to develop and study a virtual simulation through which one could learn to teach proof in high school geometry. As the design of the simulation was based on a an account of the practical rationality of mathematics teaching, developed in the context of previous projects (e.g., TheMaT I and ThEMaT II), SIMTeach also allowed us to improve that theory by exploring how a teacher’s decision to follow (or breach) a given instructional norm could depend on the context of that decision (e.g., how much time is left in class).
The project included three studies. The first was a comparison of the performance of expert and novice teachers. Through this study, we determined that expert teachers are more flexible in their adherence to recommended practices, such as discussing different approaches to solving a given problem (in this case, different proofs of the same proposition). The second study had a pre-service teacher interview a set of expert teachers as they completed the simulation. From this study, we learned that this use of the simulation provides an interesting alternative to traditional classroom observation: The preservice teacher noted that the simulation prompted discussion not only of what the expert chose to do (or would have done in their classroom), but also why they did not choose certain options (viable alternatives to what they would have done in their classroom). The preservice teacher noted that this was something that was not discussed when she had previously observed her own mentor teach. Finally, the third study—an experiment—investigated the potential of different feedback systems: one in which the user would learn from how students react to the decisions they make, as well as ones in which the user could also learn from text-screens that appear after they make a given choice and explain either what most teachers would do or how they could attend to one of their professional obligations as mathematics teachers. Our preliminary results suggest that the text-based feedback did not affect teachers’ decisions any more than the students’ reactions alone, suggesting that this additional feedback may not be necessary.
The simulation is based on realistic classroom situations, but controlled via the virtual nature of the learning environment. This learning environment allows teachers to explore and rehearse new strategies for teaching with virtual students without the higher consequences of this exploration in real classrooms. This study built on the prior work on intelligent tutors for student learning of mathematics to generalize to teacher learning. The simulation-based approach also has the potential to provide a practical framework to conduct similar studies of teaching and learning to teach in instructional situations and subjects other than the one studied here, and of learning to practice in professions other than secondary mathematics teaching.
| Title | Author(s) | Citation | Date |
|---|---|---|---|
| How can teaching simulations help us study at scale the tensions mathematics teachers have to manage when considering policy recommendations? | Herbst P, Shultz M, Bardelli, E | Herbst, P., Shultz, M., Bardelli, E., Boileau, N., & Milewski, A. (2022). How can teaching simulations help us study at scale the tensions mathematics teachers have to manage when considering policy recommendations? Educational Studies in Mathematics, 110 (1), 1-21. | 2022 |
| Subject Matter Knowledge of Geometry Needed in Tasks of Teaching: Relationship to Prior Geometry Teaching Experience | Ko I, Herbst P | Ko, I., & Herbst, P. (2020). Subject Matter Knowledge of Geometry Needed in Tasks of Teaching: Relationship to Prior Geometry Teaching Experience, Journal for Research in Mathematics Education, 51(5), 600-630. | 2020 |
| What can we learn about the differences between experts and novices from their responses to a mathematics teaching simulation? | Herbst P, Shultz M, Bardelli E, Milewski A, Boileau N | Herbst, P., Shultz, M., Bardelli, E., Milewski, A., & Boileau, N. (2019). What can we learn about the differences between experts and novices from their responses to a mathematics teaching simulation? Educational Studies in Mathematics. Springer. | 2019 |
| What simulation-based mentoring may afford: Opportunities to connect theory and practice. | Herbst P, Boileau N, Shultz M, Milewski A, Chieu V M | Herbst, P., Boileau, N., Shultz, M., Milewski, A., & Chieu, V. M. (2020). What simulation-based mentoring may afford: Opportunities to connect theory and practice. In E. Bradley (Ed.), Games and Simulations in Teacher Education (pp. 91-114). Cham, Switzerland: Springer. | 2020 |
| Can a Teaching Simulation Predict Novice and Expert Teachers’ Decision-Making? | Chieu V, Boileau N, Huisinga M, Milewski A, Herbst P | Chieu, V. M., Boileau, N., Huisinga, M., Milewski, A., and Herbst, P. (2017). Can a Teaching Simulation Predict Novice and Expert Teachers' Decision Making? Paper presented at the Annual Meeting of AERA, San Antonio, TX | 2017 |