Table of Contents

• Let’s GeT a book: Reach out to your networks by Amanda Brown
• Drawing the Line: Constructions in Hyperbolic Geometry by Steve Szydlik
• Worth a “Round” of Applause? How a Circle Arc Template Can Replace the Compass in the Learning and Teaching of Geometric Constructions by Christopher C. Tisdell
• Using FullProof in Geometry for Teachers Courses by Sharon Vestal, Orly Buchbinder, and Tuyin An
• A new working group: Engaging with the Student Learning Objectives (ESLO) by Pat Herbst
• Transformation Working Group Update by Julia St. Goar
• Teaching GeT Working Group Update by Nathaniel Miller
• GeT to Know the Community: Mara Markinson
• CALL FOR CHAPTER PROPOSALS: The GeT course: Resources and Objectives for the Geometry Courses for Teachers by Amanda Brown
• Upcoming Seminars

Let’s GeT a book: Reach out to your networks

by Amanda Brown
University of Michigan

We are excited to finally announce that we are soliciting papers for a book about GeT courses! You can find the official call for papers here: https://getapencil.org/get-writing-rfp/. This book aims to be useful for everybody interested in the undergraduate geometry courses taken by pre-service teachers. In an earlier article (https://www.gripumich.org/v3-i3-sp2022/#towards-the-development-of-a-prospectus-for-a-get-a-pencil-book), I outlined some of the possible topics in that book, the core of which includes elaborations and perspectives on a set of Student Learning Objectives (SLOs) the Teaching GeT Working Group has been working on.¹ The book is also expected to include contributions that feature instructional tasks that can be used by instructors of the course to address particular SLOs (similar to the kind of work the Transformations Working Group has been developing) and assessment tasks that can be used by instructors for gathering diagnostic, formative, or summative information about pre-service teachers’ knowledge of various SLOs. 

I am writing on behalf of my co-editors, Pat Herbst, Nat Miller, and Laura Pzdrowski, with two calls to actions: (1) consider contributing a chapter and (2) help us get the word out to others who might contribute a chapter. Our hope is that you, as a reader of GeT: The News!, will see the call for proposals as something to respond to. If you are already counting yourself out of authorship, please hear me out! We envision this book to be the kind of place where both instructors of the course and other stakeholders could contribute their perspectives. Perhaps you are an instructor who has not been involved in the development of the SLOs. Maybe you are a mathematics educator or researcher who does not teach a GeT course. Perhaps you are a high school mathematics teacher that at one time took an undergraduate geometry course. Maybe you are a mathematics consultant or teacher leader that handles professional education for inservice teachers. Maybe you are an educational administrator responsible for hiring or evaluating high school mathematics teachers. Maybe you are a mathematician, concerned with the preparation of teachers, or perhaps you are a faculty developer interested in the scholarship of teaching and learning and faculty learning communities. If any of those describes you, there may be a role for you in the book. 

If you are wondering what kind of contribution you could make, as a member of the editorial team for this book, I would like to express that we see a role for all of you—so much so that if we do not hear from you, we are committed to finding ways to seek you out (Don’t say you weren’t warned!). For one, we have already begun to discuss ways we can gather perspectives on the SLOs from a wide variety of stakeholders. This includes everyone I named above, as well as some that I did not (e.g., parents of high school geometry students, higher education administrators that rely on or staff the GeT course, designers of assessments for teachers, individuals that work at state departments of education, etc.). We are also looking for instructors who have yet been uninvolved with the development of the SLOs to share their perspectives on the SLOs. We also think that there are some clear opportunities for others, such as mathematics educators, professional development providers, high school mathematics teachers, mathematicians, and mathematics education researchers, to get involved in making submissions–by offering perspectives about the SLOs, submitting instructional materials connected to the SLOs, or suggesting ways of expanding the circle of stewards of geometry for teachers

Next, in order to convince the as-yet-unconvinced to make a contribution to the call for proposals, it might be important for me to say more about what the core kinds of contributions might look like. As noted above, we see this book as a place where a wide variety of stakeholders could share their perspectives about the SLOs. The Working Group that first proposed the SLOs envisioned them as a living document that might undergo revisions as the original version were exposed to a wider and wider set of perspectives. In fact, we have already gotten started. We are currently in the process of convening a new working group, including GeT instructors who had not been involved in the drafting of SLOs and experienced secondary geometry teachers who know what the demands of teaching high school geometry are, in order to start gathering complementary perspectives. We hope that a team of individuals from that group will contribute a chapter or two. If you are interested in learning more about the SLOs, you can view them at the following website: https://getapencil.org/student-learning-objectives/. A plethora of previously-published articles in GeT: The News! provide examples of what it might look like to take a perspective on one or more of the SLOs (see A GeT Course “Classic”: The Euclidean Archetype in Vol. 1 Iss. 1, Understanding Student Thinking on Transformation Congruence Proofs in Vol. 2 Iss. 1, On mathematical knowledge for teaching geometry and the SLOs: A Reflection in Vol. 3 Iss. 3; Proof: The Heart of the Geometry for Teachers Course in Vol. 2 Iss. 1). 

In addition to seeking perspectives on the SLOs, we are thinking the book could be a place for a variety of individuals to share materials–whether they be activities or assessments–that could serve as resources for instructors, including lessons, assessment items, and assignments targeting specific objectives from among the SLOs. These contributions could be drawn from a variety of sources—including materials that have been designed and used with individuals different from GeT students (e.g., materials drawn from work with inservice teachers in a professional development context, or materials drawn from high school geometry classrooms). Examples of these kinds of contributions abound in our prior newsletters, and many of those articles may be reasonable starts for contributions (see When More is Not Always Better: On How NOT to Approach an Elementary Construction in Vol. 1, Iss. 3, The Geometry of Sunlight in Vol. 2, Iss. 3; People and Clubs: An Axiomatic System in Vol. 2, Iss. 2, Exploration, Construction, and Proof as Resources for Teaching Geometry Through Problems in Vol. 1, Iss. 2)

As one of the editors of this volume, I realize the vision I have outlined here—to gather a wide variety of stakeholders to make contributions—is ambitious and something the editors will not be able to achieve alone. For these reasons, we are looking to you to help us accomplish this vision by spreading the word about this call for proposals. In order to partner with us on recruiting individuals that will expand both the number and type of contributions, it might be helpful for me to further elaborate on the agenda I describe above with some examples. In these examples, I will intentionally focus on the types of contributions that might be made uniquely by stakeholders different from GeT instructors and that you may have in your networks. I focus on these examples because I believe that without some kind of recruitment action on our part, it is unlikely we will receive the kinds of contributions I describe, and my hope is that by providing these examples I can expand our collective thinking about what different kinds of submissions might contribute.

One kind of stakeholder, distinct from GeT instructors, is an individual employed at an institution that has responsibility to procure or design assessments for gauging professional knowledge held by secondary mathematics teachers (e.g., a mathematics consultant at a state department of education or a psychometrician at a test development organization). This kind of individual might be uniquely positioned to contribute a chapter that makes connections from the SLOs to current assessment instruments used to assess secondary mathematics teachers’ professional knowledge. We could envision, for example, a conceptual contribution from such an individual that identifies connections and possible disconnections between the SLOs and the underlying objectives assessed within widely-recognized assessments. This kind of individual might also be uniquely positioned to contribute a chapter that provides information about what is known about teachers’ professional knowledge related to the teaching and learning of secondary geometry. Both of these contributions could go a long way in helping inform the work of GeT instructors related to the SLOs.

A different kind of stakeholder is an individual serving as a high school geometry teacher. One way we could envision a high school teacher contributing their unique perspective is by offering a reflection on the kinds of challenges they experienced in transitioning from their undergraduate experience to the teaching of geometry or the challenges they have seen newer colleagues experiencing when they are assigned to teach geometry for the first time. For example, in such a reflection, a teacher could share about the kinds of experiences they had in the undergraduate course and how those experiences did or did not prepare them for their work teaching high school geometry. This kind of reflection can help shine a light on the importance of various SLOs as well as potentially identifying other learning objectives and pondering whether they are captured among the current SLOs or might need to be considered for inclusion. Another way we could envision such a person making a contribution is by writing case studies about how high school students grapple with different kinds of mathematical tasks common to the high school geometry curriculum and the way a teacher uses their knowledge of geometry to support students’ learning. For example, a high school teacher might elect to implement one or more of the tasks that have been featured in the GeT: The News! (e.g.,Teaching for Understanding in Vol. 1, Iss, 2, Illustrating a modeling approach to high school geometry: The pool problem in Vol. 2, Iss. 3, or Reflecting on the SunRule as a Multiplication Model in Vol. 2, Iss. 3,) and share anonymized artifacts illustrating how high school students think about this task or how they responded to those ways of thinking. Both kinds of contributions can be valuable resources for GeT instructors in terms of shaping instruction and offering alternative perspectives on the SLOs.

In offering these examples, my hope is that I have stretched your imagination about what various contributions to the recent call for proposals might look like. I also hope that these examples have supported you in developing some of your own ideas about ways we can think more broadly about the contributions for this book—and that you will share those ideas back with us (either in the form of a proposal or through a conversation–or both). 

Prior to closing, I want to use this opportunity to invite you all to an online conversation with the co-editors. It is scheduled for October 21, 2:00 PM ET. You can find more information about this event here.

With that, I will close with a call for two community-wide actions:

• For everyone reading this, please share either this article or the call for proposals with others. We ask that, as you do, you think broadly about the variety of individuals that are in your network–the diversity of roles, expertise, perspectives–whose contributions would be beneficial to our collective.

• For those of you reading this that have not yet considered making a contribution, please reconsider. If you have an idea for a contribution but are unsure how it might fit, feel free to reach out to me or another of the co-editors. We would love to grab coffee with any of you–in person or virtually–to discuss your ideas and give feedback. 

_________________________

¹See https://getapencil.org/student-learning-objectives/

Suggested Citation

Brown A. (2022, October). Let’s GeT a book: Reach out to your networks. GeT: The News!, 4(1). https://www.gripumich.org/v4-i1-f2022/#lets-get-a-book-reach-out-to-your-networks

Drawing the Line: Constructions in Hyperbolic Geometry

by Steve Szydlik
University of Wisconsin Oshkosh

Introduction: Hyperbolic Geometry and Its Models

Hyperbolic geometry resides in a unique but challenging position within the GeT curriculum, providing powerful opportunities for an instructor to enrich student understanding of geometry. First, it provides experiences with a significant axiom system distinct from Euclidean geometry. Second, by changing a single axiom (the parallel postulate), it provides a meaningful contrast to Euclidean geometry, thereby offering worthwhile insights into that more familiar world. Third, it provides visible support for a variety of essential Student Learning Outcomes (SLOs). Finally, it adds historical context to the lively story of 2000 years of geometry, which began with Euclid. 

However, hyperbolic geometry presents a conundrum for GeT instructors. It can be intimidating to both students and instructors because it contains strange and hard-to-visualize theorems. How can we have parallel lines that have but one common perpendicular? What do limiting parallel rays look like? How can there be more than two lines passing through a point parallel to a third line? Hyperbolic geometry can appear esoteric, unreal, and unimportant when viewed as an abstract axiom system. A GeT instructor, particularly one without experience in hyperbolic geometry, might naturally choose to downplay the subject in the classroom, especially given the course’s many other essential topics.

For me, the solution to this challenge entails combining several aspects of the GeT course curriculum: axiomatic models of hyperbolic geometry, Euclidean straightedge and compass constructions, and dynamic geometry software. These three ingredients combine to provide a powerful pedagogical tool for understanding hyperbolic geometry.

Models of an axiom system are interpretations of essential undefined terms that satisfy the axioms. In the case of hyperbolic geometry, these terms include “point,” “line,” and “congruence.” When interpreted properly in the models, all of the axioms of hyperbolic geometry are satisfied, particularly the hyperbolic axiom: there exists a line ℓ and a point P not on ℓ such that there are at least two lines through P parallel to ℓ. A logical consequence is that in models of hyperbolic geometry, all of its theorems are valid. The models help us make sense of these theorems by allowing us to experience hyperbolic worlds in concrete ways. As such, we can use them as laboratories to explore conjectures and visualize theorems.

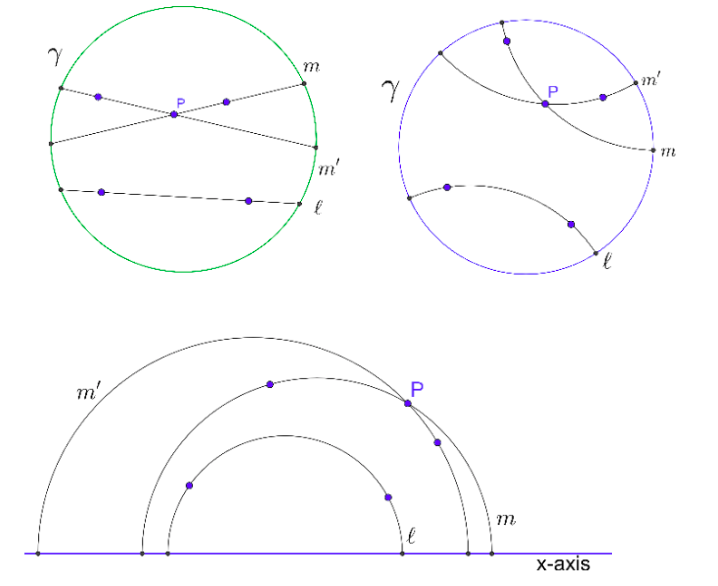
The three models we briefly consider here are the Beltrami-Klein disk, the Poincaré disk, and the Poincaré half-plane:

• In the Beltrami-Klein disk (K-disk henceforward), we interpret “points” to mean points interior to a fixed circle γ. “Lines” are open chords of γ.
• In the Poincaré disk (P-disk), “points” are points interior to a fixed circle γ, while typical “lines” are open arcs of circles, interior to γ but orthogonal to γ.
• In the Poincaré half-plane (Half-plane), “points” are points above the x-axis in the usual Euclidean plane, while typical “lines” are open semicircles above the x-axis, centered on the x-axis.

If we define a distance metric and “congruence” in terms of that metric, then each of these interpretations satisfies the axioms for hyperbolic geometry, making it a model. In each of the models, the hyperbolic axiom, with its multiple parallels, becomes transparent (see Figure 1, or a dynamic version at https://tinyurl.com/szydlik22).

Euclidean and Hyperbolic Constructions and the Role of Dynamic Geometry Software

Classical straightedge and compass constructions have been a part of the geometry curriculum since the time of Euclid. Their pedagogical utility is clear; they encourage the use of precise mathematical language by the constructor. They drive mathematical arguments; “How do you know that your construction works?” is a natural question to ask. Constructions provide students with experience in problem-solving and preservice teachers with opportunities to practice the curriculum they can expect to teach (see CCSSM, 2010). Indeed one of the essential SLOs is that students should “be able to carry out basic Euclidean constructions and justify their correctness” (SLO 8). Early in the GeT course that I teach, we spend a day or so developing basic Euclidean constructions and arguing their correctness. We bisect and copy angles, raise and drop perpendiculars, construct parallels and perpendicular bisectors, and, as a homework assignment, construct a circle passing through three noncollinear points.

The Euclidean constructions and the hyperbolic models are inextricably linked. Because hyperbolic models are defined as objects in the Euclidean plane, any geometric structures in the models are entirely Euclidean. The interplay between the models and Euclidean constructions is bidirectional. We rely on Euclidean constructions when we wish to visualize a hyperbolic theorem in one of the models. On the other hand, when we execute a construction of a hyperbolic object in one of the models, we enhance our understanding of Euclidean constructions. For example, consider the challenge of constructing a hyperbolic line through two points in the half-plane. In Euclidean terms, this amounts to constructing the semicircle centered on the x-axis that passes through two points A and B that are above the x-axis. The steps required to complete this construction include finding the point O where the perpendicular bisector of segment AB meets the x-axis and constructing the circle centered at O that passes through A (and B). This is not a particularly difficult problem, but one can see that it does require problem-solving on the part of the students, as well as a facility with basic Euclidean constructions (see Figure 2, or the dynamic geometry version at https://tinyurl.com/szydlik22).

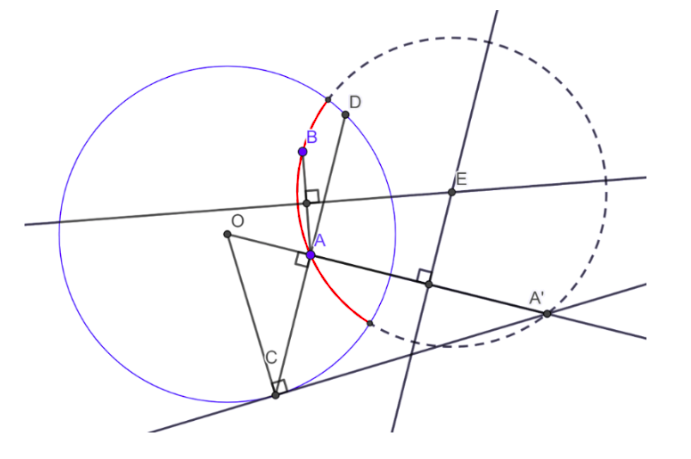
Of course, constructions in hyperbolic geometry may require a significant number of steps. Consider the process of constructing the “line” through two points, A and B, in the P-disk. This involves finding the inverse point A’ for A, then constructing the circle passing through A, B, and A’ (see Figure 3, or the dynamic geometry version at https://tinyurl.com/szydlik22). This construction necessitates the following Euclidean steps:

1. Let O be the center of the given Poincaré disk γ. Construct ray OA.
2. Construct the line through A perpendicular to OA. Let B and C be the intersection points of this perpendicular with circle γ.
3. Construct the tangent to γ at C (or D). This is the line perpendicular to OC at C.
4. Let A’ be the intersection of this tangent and ray OA. A’ is the inverse of A relative to circle γ.
5. Construct the perpendicular bisectors of segments AA’ and AB. Let E be the intersection of those perpendicular bisectors.
6. Construct the circle centered at E with radius EA. By construction, this circle passes through A’ and B as well. The part of this circle interior to γ is our Poincaré line through A and B.

The steps in the construction of a P-disk line are entirely Euclidean, and carrying out this construction will support a student’s understanding of the relevant Euclidean constructions. However, completing these steps every time we desire a Poincaré line is time-consuming and can distract from a higher-order task that might use that line. This is where dynamic geometry environments (DGEs) such as GeoGebra offer a convenient solution. GeoGebra automates basic Euclidean constructions, eliminating much of the low-level straightedge and compass strokes (note that while GeoGebra is my DGE of choice, many other options are available, including Geometer’s Sketchpad, Cinderella, and Cabri).

One especially useful feature of GeoGebra (and other DGEs) is the ability to create custom “tools,” or macros that automate a series of steps in a construction. For example, rather than constructing all of the chords, perpendiculars, tangents, and circles required to create a hyperbolic line in the P-disk, a user might use a GeoGebra “Poincaré Line” tool to simply plot two points in the disk, with the tool automatically drawing the required circle arc through the two points.

The Hyperbolic Toolbox

GeoGebra offers quick access to construction tools for Euclidean geometry, and I find it useful for my students to have access to similar tools in the hyperbolic models, beyond just the hyperbolic line tool described above. So, together we develop a toolbox of “essential” hyperbolic constructions in each model. We have tools to construct hyperbolic segments, rays, and lines, bisect angles, raise and drop perpendiculars, construct circles, and measure lengths and angles. Measuring is not a construction, of course, but automating the measurement process in our models is helpful, especially when we want to confirm congruence of segments or angles. With this full suite of tools, we can execute complex hyperbolic constructions in our models. Moreover, utilizing the dynamic nature of the software, we can drag the free points in any construction and see the effects on subsequent constructions. This allows us to bring the full power of the constructions to bear.

In my GeT course, we apply the hyperbolic tools in three distinct ways. First, though I provide most of the tools to students, I ask them to develop some of the tools themselves. Specifically, students have the facility to develop tools to construct lines in each of the models, especially if provided with some necessary background in the case of the Poincaré disk. Raising perpendiculars is both an accessible task and a great problem-solving challenge for students.

Second, we use the construction tools as building blocks for more extensive hyperbolic constructions. One exercise is to explore whether common Euclidean objects exist in hyperbolic geometry and if so, what properties they might possess. For example, while it is easy to construct a rectangle in Euclidean geometry, can we do the same in hyperbolic geometry? Where does the construction go wrong? What about a rhombus? The tools offer us opportunities to better understand the history of geometry as well, specifically in the attempts to prove the parallel postulate. Each such attempt to prove Euclid’s fifth postulate was doomed to failure. While identifying a flaw can be challenging in the abstract, carrying out the constructions in a hyperbolic model can be illuminating. In particular, the flaw in each proof will always appear in our hyperbolic models. Figure 4 illustrates one proof attempt by Farkas Bolyai (see Greenberg, 2008, p. 229). The dynamic sketch at https://tinyurl.com/szydlik22 provides some of the flavor of such an activity, though the students would typically carry out all the necessary constructions, rather than having them provided in “checkbox” form.

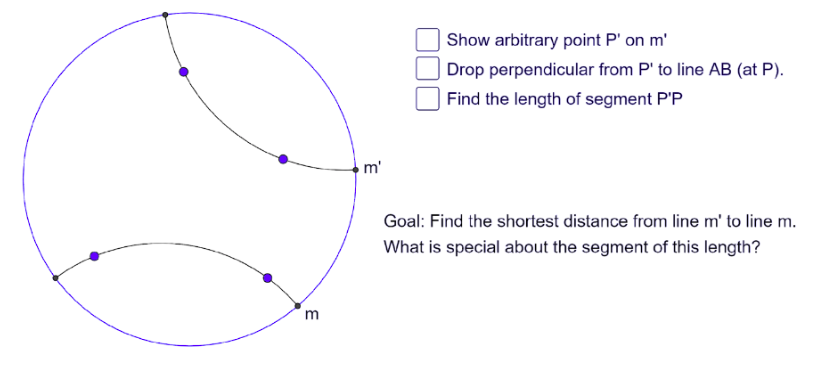
Finally, we use our hyperbolic toolbox to motivate and to visualize the major theorems in hyperbolic geometry. For example, among the strangest ideas in that world is that parallel lines have at most one common perpendicular, and when it exists, the common perpendicular segment offers the shortest distance between the parallel lines. Giving students parallel lines in one of the models, having them find the (hyperbolic) shortest segment between the lines, and identifying the key properties of that segment offers rich opportunities for exploration (see Figure 4, or the dynamic sketch at https://tinyurl.com/szydlik22).

Supporting Student Learning Outcomes

Hyperbolic constructions offer opportunities for an instructor to address many of the essential SLOs in a GeT course in meaningful ways while also supporting teaching practices endorsed by the NCTM (2020) and others (e.g. Abell et al., 2017). The connections to several of these SLOs are clear: working within the models using DGE helps emerging teachers to develop their abilities to “[c]ompare Euclidean geometry to other geometries such as hyperbolic or spherical geometry” (SLO 9), to “carry out basic Euclidean constructions and justify their correctness” (SLO 8), and to “effectively use technologies to explore geometry and develop understanding of geometric relationships” (SLO 6). Moreover, when exploring hyperbolic geometry and constructions in general, students naturally gain experience in proof (SLO 1). With the availability of the hyperbolic toolbox, additional activities become feasible that support student development addressing other learning outcomes. For example, working with models can increase student understanding of the role of axioms in geometry (SLO 4). Asking students to find flaws in proofs of the parallel postulate enriches their knowledge of the history of geometry and strengthens their appreciation for Euclid (SLO 7). Indeed, though many prospective teachers may never encounter hyperbolic geometry in the curricula that they teach, exposing them to the beauty of hyperbolic geometry is, nevertheless, a worthwhile experience.

Resources

There are a wide variety of resources for instructors interested in further exploring dynamic constructions in the hyperbolic models. On my personal website, you can find GeoGebra files that include the full suite of tools associated with each of the three models discussed above. There, you can also access links to GeoGebra sketches that describe the development of those tools as well as examples of ways to use the tools in the classroom. Alternatively, a search through the activities at geogebra.org, perhaps using the names of the models as keywords, produces a wealth of resources. For non-GeoGebra users, the Cinderella software includes built-in construction tools for the Poincaré disk, and NonEuclid offers a standalone package of Poincaré disk tools as well. 

References

Abell, M., Braddy, L., Ensley, D, Ludwig, L., & Soto-Johnson, H. (2017). Instructional practices guide. Mathematical Association of America. 

GeT: A Pencil. (2022). Student Learning Outcomes. Available at https://getapencil.org/student-learning-objectives/. 

Greenberg, M. J. (2008). Euclidean and non-Euclidean geometries: Development and history (4th ed.). Freeman.

National Governors Association Center for Best Practices & Council of Chief State School Officers. (2010). Common Core State Standards for Mathematics [CCSSM]. Washington, DC: Authors. Available at http://www.corestandards.org/Math/. 

National Council of Teachers of Mathematics [NCTM] (2020). Standards for the preparation of secondary mathematics teachers. Available at https://www.nctm.org/uploadedFiles/Standards_and_Positions/NCTM_Secondary_2020_Final.pdf. 

Suggested Citation

Szydlik S. (2022, October). Drawing the Line: Constructions in Hyperbolic Geometry. GeT: The News!, 4(1). https://www.gripumich.org/v4-i1-f2022/#drawing-the-line-constructions-in-hyperbolic-geometry

Worth a “Round” of Applause? How a Circle Arc Template Can Replace the Compass in the Learning and Teaching of Geometric Constructions

Christopher C. Tisdell
The University of New South Wales, UNSW, Sydney, Australia

What types of physical tools do you expect students and educators to use in the learning and teaching of geometric constructions? Although Euclid’s Elements remains silent on prescribing particular tools, do you expect learners and teachers to keep to the so-called Platonic restrictions? That is, are they limited to exploring geometric constructions with an unmarked straightedge and compass only? If so, why? Certainly, the compass and straightedge are the most well-known set of tools in the learning and teaching of geometry, with learners using them over millennia, and thus our connection to these tools is partially driven by tradition and familiarity (Albrecht, 1952). In addition, there are some high-school curricula that impose the use of these two tools alone, and thus our alignment with the Platonic restriction is partly driven by such curriculum constraints.

However, there are non-trivial problems with students and teachers using compasses as a practical tool of geometry education. For example, many learners (especially younger learners) find compasses difficult to operate from a kinesiological perspective due to the fine motor skills required. We all have experienced the unpleasantness of a compass slipping when being rotated and producing wobbly and unsatisfying arcs. As a result, the compass as a drawing tool is somewhat inefficient, and the produced drawings can be frustratingly inaccurate. Furthermore, traditional compasses pose safety risks as they can be weaponized by using the pointed leg. Thus, there is a need to reconsider the compass and to offer an alternative in the learning and teaching of geometric constructions. 

The purpose of this short article is to partially respond to the above need by discussing the potential of a circle arc template to replace the compass in the learning and teaching of geometric constructions within schools. I will discuss a circle arc template and, through a reflection on a series of questions I have received, probe its design and capabilities against a traditional compass. As such, I hope this article will be of value to the GeT: A Pencil community by providing an alternative construct that can be explored in the classroom.

Figure 1 is a photograph of a circle arc template. Essentially, it is a clear piece of polycarbonate with sections removed from it to make a PAC-MAN™ type shape. There is a positionable center point in the center of the template. Learners can place the center point of the template over a given point on the page and trace around the inside to form an arc of fixed radius up to 300 degrees. Learners operate the circle arc template in a familiar way much like a ruler; that is, one hand keeps the template steady, and the other hand holds the pencil to trace out an arc. 

Let me reflect on and respond to some of the most common questions I have received about the template when I have shown it to colleagues, teachers and students: 

Q: Learners cannot adjust the radius of the circle arc template tool. Is that a problem?

A: No. All of the Euclidean constructions can be performed under this restriction together with an unmarked straightedge. In fact, the idea of the fixed radius of the template is similar to the concept of a “rusty compass” in geometric construction, where the angle of the opening of legs of the compass cannot be adjusted (and thus only circles and arcs with a fixed radius can be drawn). The capability of a rusty compass and an unmarked straightedge to act as sufficient tools for all of Euclid’s constructions has been known for more than 450 years (Mackay, 1886).  

Q: Learners cannot draw a full circle with the circle arc template tool. Is that a problem?

A: No. All of the Euclidean constructions can be performed under this restriction together with an unmarked straightedge. More than a century ago, Severi (1904) showed that if students have an arc of any circle–however short in arc length–together with its centre point and an unmarked straightedge, then all of Euclid’s constructions are possible. Observe that the circle arc template in Figure 1 satisfies Severi’s conditions when used in conjunction with an unmarked straightedge.

Q: When using the circle arc template, are the steps in constructions the same as when a traditional compass is used?

A: Not necessarily. In simple constructions such as bisecting a given angle, the steps can be identical. However, in other simple circumstances, such as constructing an equilateral triangle on a given line segment, the steps are different and owe much of their strategies to those associated with the rusty compass. Thus, there are some familiarities and some differences, and employing such a circle arc template has the ability to foster students thinking beyond traditional problem solving pathways. Some basic constructions with the circle arc template can be found in this YouTube playlist (Tisdell, 2021).

Q: Why focus on developing physical tools and not on digital software for the learning and teaching of geometric construction?  

A: Software for the learning and teaching of geometric construction is becoming more prevalent in schools. However, access to digital resources is not always readily available due to cost, complexity of the infrastructure and the challenge of connecting with it. Furthermore, in my opinion, the choice between the physical and the digital forms a false dichotomy, as they can be developed together. For example, what roles and possibilities could a “digital” circle arc template or rusty compass have within educational geometry software? 

Q: How have students reacted to using and learning with the circle arc template?

A: Many students have expressed their familiarity with using templates as a drawing tool and communicated their ease of use to me. Especially in younger learners (ages 7-11), I have observed much more accuracy in their geometrical drawings using the circle arc template than when using a traditional compass. This suggests that using a circle arc template enables learners to devote more attention to the relationships between geometric objects and principles rather than concentrating on producing an accurate drawing with a traditional compass.  

Q: Where can I find out more?

A: In addition to the YouTube playlist (Tisdell, 2022) mentioned above, there is a recent publication (Tisdell and Bee Olmedo, 2022) where the ideas herein are expanded upon.

Although I am excited by the circle arc tool and secretly hope it deserves a “round” of applause, I would also love to hear your thoughts, ideas, criticisms, and experiences in using non-traditional tools for the learning and teaching of geometric constructions. I welcome any questions and feedback from the GeT: A Pencil community. The circle arc template is undergoing the patenting process. 

References

Albrecht, W. A. J. A Critical And Historical Study Of The Role Of Ruler And Compass Constructions In The Teaching Of High School Geometry In The United States, PhD Thesis, 1952, The Ohio State University, Ann Arbor. https://etd.ohiolink.edu/apexprod/rws_etd/send_file/send?accession=osu148639654226706&disposition=inline 

Mackay, J. S. Solutions of Euclid’s Problems, with a rule and one fixed aperture of the compasses, by the Italian geometers of the sixteenth century. Proceedings of the Edinburgh Mathematical Society, 1886, 5: 2‒22. https://doi.org/10.1017/S0013091500001334 

Severi, F. Sui problemi determinati risolubili colla riga e col compasso (Estratto da una Lettera al Prof. F. Enriques). Rendiconti del Circolo matematico di Palermo, 1904, 18(1): 256‒259. https://doi.org/10.1007/BF03014102 

Tisdell, C. C. Learning geometry with a circle arc template. YouTube Playlist, 2022. https://www.youtube.com/playlist?list=PLGCj8f6sgswmZBXkjWXcwQQcErW1QUS8A 

Tisdell, C. C. and Bee Olmedo, D., Beyond the compass: Exploring geometric constructions via a circle arc template and a straightedge. STEM Education, 2022, 2(1): 1-36. https://www.aimsciences.org/article/doi/10.3934/steme.2022001 

Suggested Citation

Tisdell, C. (2022, October). Worth a “Round” of Applause? How a Circle Arc Template Can Replace the Compass in the Learning and Teaching of Geometric Constructions. GeT: The News!, 4(1). https://www.gripumich.org/v4-i1-f2022/#worth-a-round-of-applause-how-a-circle-arc-template-can-replace-the-compass-in-the-learning-and-teaching-of-geometric-constructions

Using FullProof in Geometry for Teachers Courses

by Sharon Vestal
South Dakota State University

Orly Buchbinder
University of New Hampshire

Tuyin An
Georgia Southern University

Introduction 

Proof-writing is a core disciplinary practice of mathematicians and a crucial skill of all mathematics majors and future mathematics teachers. Developing students’ facility and comfort with proofs is an important objective of undergraduate Geometry for Teachers (GeT) courses (An et al., in press; Grover & Connor, 2000). GeT courses cultivate robust and flexible knowledge and skills such as writing, analyzing, and critiquing proofs that allow prospective secondary teachers (PSTs) to support their students’ learning of geometry (AMTE, 2017; González & Herbst, 2006). 

Technology plays an important role in supporting PSTs’ engagement with proof. Dynamic Geometry Environments like GeoGebra, Geometer’s Sketchpad, and others provide opportunities to explore geometrical properties and make and test conjectures (Jones, 2000; Mariotti & Baccaglini-Frank, 2018). These tools have been beneficial for fostering PSTs’ attitudes towards proof and comfort with proof and teaching it to secondary students (Abdelfatah, 2011; Kardelen & Menekse, 2017). However, they offer little support for writing a deductive proof of a conjecture or a theorem. 

With the advancements in artificial intelligence and machine learning, new tools have been created that support automated and interactive proof writing and verification (e.g., Lodder et al., 2021; Matsuda & VanLehn, 2005; Wang & Su, 2017). FullProof is a software developed to advance students’ proof-writing skills in Euclidean Geometry. Using FullProof, students write a step-by-step two-column proof, using an interactive diagram, an equation editor, and a library of theorems/postulates and definitions. The software checks the proof and provides feedback and/or hints to improve their work. 

Our project aimed to explore the potential benefits of FullProof for GeT students; our research question was: How and to what extent does engaging GeT students with the FullProof software affect their competence with, and beliefs about geometry proofs? 

Methods

Research Setting

In Fall 2021, we conducted an exploratory study by integrating FullProof into our GeT courses, which all have a large portion of the curriculum devoted to Euclidean Geometry from a synthetic perspective. Two GeT courses had a mixed student body of PSTs and STEM majors; one GeT course only had PSTs. Thirty students agreed to participate in the study across the three GeT courses. 

FullProof served as a support system for writing proofs in class and homework. Each instructor used about 20 proof problems from FullProof ‘s collection, on a variety of topics, at three levels of difficulty: high, medium, and low. Figure 1 shows a screen capture of a sample problem (medium difficulty) about triangle midsegments. 

Each element in the figure can be highlighted or marked when pointed to, and auxiliary lines can be added as needed (Fig. 2). The right side of Figure 1 shows the givens and the statement to be proven. The students write the proof by typing statements in the numbered lines and justifying them. The justifications can be searched by keywords or by browsing the FullProof justifications library. 

At any point in the process, the students may ask for a hint by clicking the hint button. The system will produce hints in order of increased specificity from a vague “try using triangle congruence,” to suggesting a certain theorem to use, to proposing a particular step–like “try proving ∆BFO ≅ ∆EGO.”

Once finished, the students click the “check” button, and in a few seconds, the algorithm checks the proof and provides feedback. Correct proof lines get a green check mark. Incorrect or partially correct lines are marked down with an explanation of the mistake. Figure 2 shows a solution with two mistakes – a missing proof step and a missing justification for the last step. Clicking on the notifications will show a student what the missing step was (here ∆BFO ≅∆EGO by angle-side-angle theorem) and how many points were deducted for each mistake.

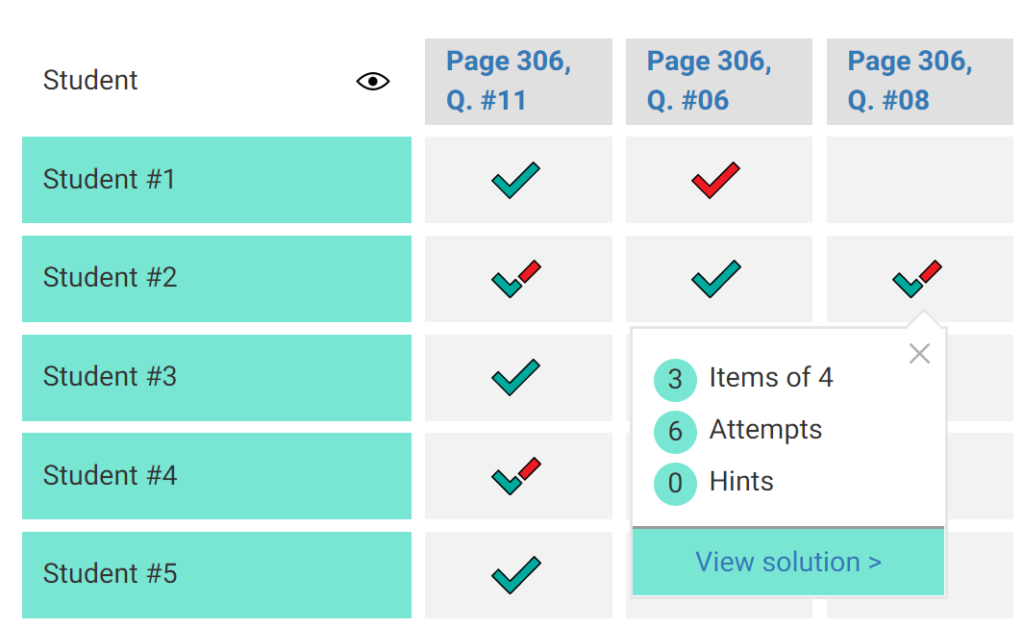
Clicking the “try again” button allows a student to improve their work, resubmit, and receive more feedback. The number of hints and submission attempts is unlimited (except for the test mode), allowing the students to achieve a perfect score eventually. The information about the number of hints and submission attempts is stored in the system and is available on the instructor’s interface (Fig. 3). 

Data Collection and Analysis

To respond to our research question, we administered pre- and post-surveys via Qualtrics, which took about 20 minutes to complete. Most questions were Likert-type on mathematical identity and comfort level with writing proofs. The items were adapted from the literature (e.g., Kaspersen & Ytterhaug, 2020; Stylianou et al., 2015). Here, we focus on responses to the bolded open-ended questions, developed by the researchers for the post-survey, about student perceptions of FullProof (Fig. 4). We analyzed the data qualitatively, using open coding and thematic analysis (Patton, 2002) to reveal recurring themes in students’ responses, specifically the types of positive and negative appraisals about using FullProof. To capture all themes, comments that included multiple ideas were coded multiple times.  

Figure 4. Open-ended Items from the FullProof Survey.

Student Appraisals of the FullProof Platform 

The analysis of the positive and negative appraisals in students’ written comments on the post-survey revealed three main themes that emerged from the 61 positive appraisals of FullProof identified across all seven open-ended questions. The largest category (41%) described the affordances of FullProof for supporting students’ writing and understanding of proofs, including the searchability of reasons for proof steps, clear structure that supports communication and comprehension, interactive feedback, and the ability to pursue multiple solution paths. One student wrote, “FullProof has helped me a lot when writing proofs. I like how I can search for reasons if I am not completely sure about a reason/theorem.” Another student wrote, “FullProof made writing proofs easier not only to write, but also to understand.”  

The second theme (26%) described the advantageous technical features of FullProof, such as hints which help them move forward if stuck, interactive feedback pointing to errors, visual clarity, and color-coded elements of a diagram. The third theme (25%) described the affordances of FullProof as a pedagogical tool for teaching others. In this theme, the participants highlighted the elements of the software that would support them as teachers in the geometry classroom. Students wrote that FullProof presents “a good instructional strategy to implement in the classroom,” and the platform “makes it easier for the students to see what their errors were.” 

The 23 negative appraisals constituted 29% of the codes and were distributed rather uniformly across five themes. The main critique (7 out of 23 appraisals, 30%) concerned the discrepancy between the wording of the theorems in the FullProof platform and in class. For example, FullProof does not have the angle-angle-side triangle congruence theorem, so students need to complete an extra step and use the angle-side-angle congruence theorem. Another category of critiques (22%) described the variation in standards of rigor employed by FullProof vs. classroom instructors. The students wrote that the software allowed them to skip steps and earn full points for their solution, while they were aware that their instructor would have likely deducted points. One student wrote: “It allows some things to slide, that should be wrong.” 

Additional patterns emerged from the analysis of specific survey questions. When asked, “Has FullProof changed the way you write proofs,” the responses split almost evenly between yes and no. But in the written comments clarifying the forced choice, 83% of comments were positive regarding FullProof. A similar pattern was observed in the question: “How has FullProof changed your understanding of reasoning and proof?” Sixty-one percent of students wrote that FullProof positively influenced their understanding, but interestingly, 91% of their comments contained some specific description of the positive effect of FullProof. When asked, “Would you use FullProof in your future classrooms,” 14 out of 17 students who answered this question responded positively citing the advantages of the platform. When asked to describe some of the successes and challenges students had with FullProof, 65% of participants’ comments described challenges, as opposed to 35% that described successes. However, the overall impressions of FullProof were very positive with 72% of comments containing positive reviews of the tool. These results show positive appraisals of the FullProof platform outweigh the critiques of the tool. 

Discussion

Our quasi-experimental research design has several limitations. Due to the lack of randomization and a single experimental group, potential confounding variables were not controlled (Johnson & Christensen, 2012). Each course was taught by a different instructor, with no common curriculum or textbook across the institutions. There was also some variation in how instructors used FullProof in their courses, with one institution teaching in a hybrid mode due to the Covid-19 pandemic. Because of these variations, our study design emulates natural conditions of how instructors might use any technological tool within the unique constraints of their institutional environments. Against this backdrop, we find it encouraging that the qualitative analysis showed more positive appraisals (69) than negative appraisals (27) about using the platform which indicates the overall positive impact of using FullProof in the GeT course. Moreover, since our analyses did not distinguish between PSTs and other STEM majors, the observed potential advantages of FullProof may apply to all GeT students.

As instructors, we note several advantages to using FullProof in our GeT courses. The availability of multiple proof problems at various difficulty levels and the feedback provided by the FullProof platform allowed assigning a greater number of challenging problems, compared to the past. Since FullProof offers hints and multiple submissions, students were able to get assistance from the software itself. However, this also presented a challenge; some students abused this functionality to get hints on every proof step. In the future, we plan to limit the number of hints and submission attempts. Another limitation of FullProof was the observed lack of rigor in its auto-grading system. We observed cases that FullProof issued students three stars (excellent) on their proof when certain necessary proof steps were missing or the reasons were not matching the statements. This limitation makes FullProof more suitable as a learning tool instead of a formal assessment tool. However, the discrepancies in the grading standards between the software and the instructor can be leveraged to engage students in analyzing and critiquing proofs, which is an important learning objective of GeT courses.

Based on what we learned from the pilot study, we modified the research design and are currently collecting the second round of data in our GeT courses. Some changes include shortening the surveys and assigning a few common FullProof problems across courses. While we feel FullProof is overall an efficient tool in facilitating the learning of proof, we want to further study how and to what extent it improves GeT students’ proof learning experience.    

References

Abdelfatah, H. (2011). A story-based dynamic geometry approach to improve attitudes toward geometry and geometric proof. ZDM-Mathematics Education 43, 441–450. doi.org/10.1007/s11858-011-0341-6

Association of Mathematics Teacher Educators. (2017). Standards for Preparing Teachers of Mathematics. https://amte.net/standards   

An, T., Boyce, S., Brown, A., Buchbinder, O., Cohen, S., Dumitrascu, D., Escuadro, H., Herbst, P., Ion, M., Krupa, E., Miller, N., Pyzdrowski, L., J., Sears, R., St. Goar, J., Szydlik, S., Vestal, S. (in press). (Toward) Essential student learning objectives for teaching geometry to secondary pre-service teachers. AMTE Professional Book Series, Volume 5: Reflection on Past, Present and Future: Paving the Way for the Future of Mathematics Teacher Education.

González, G. and Herbst, P. (2006). Competing arguments for the geometry course: Why were American high school students supposed to study geometry in the twentieth century? International Journal for the History of Mathematics Education, 1(1), 7-33.

Grover, B., & Connor, J. (2000). Characteristics of the College Geometry Course. Journal of Mathematics Teacher Education, 3, 47–67.

Hanna, G., Reid, D. A., & De Villiers, M. (Eds.). (2019). Proof Technology in Mathematics Research and Teaching. Springer International Publishing.

Johnson, B., & Christensen, L. (2012). Educational research: Quantitative, qualitative, and mixed approaches (4th ed.). SAGE Publications, Inc. 

Jones, K. (2000). Providing a foundation for deductive reasoning: Students’ interpretations when using dynamic geometry software and their evolving mathematical explanations. Educational Studies in Mathematics, 44(1), 55–85.

Kardelen, G., & Menekse, S. T. B. (2017). Views of pre-service teachers following teaching experience on use of dynamic geometry software. Educational Research and Reviews, 12(24), 1208-1219.

Kaspersen, E., & Ytterhaug, B. O. (2020). Measuring mathematical identity in lower secondary school. International Journal of Educational Research, 103, 101620. https://doi.org/10.1016/j.ijer.2020.101620

Lodder, J., Heeren, B., Jeuring, J., & Neijenhuis, W. (2021). Generation and Use of Hints and Feedback in a Hilbert-Style Axiomatic Proof Tutor. International Journal of Artificial Intelligence in Education, 31(1), 99-133. 

Mariotti, M. A., & Baccaglini-Frank, A. (2018). Developing the Mathematical Eye Through Problem-Solving in a Dynamic Geometry Environment. In Broadening the Scope of Research on Mathematical Problem Solving (pp. 153-176). Springer.

Matsuda, N., & VanLehn, K. (2005). Advanced Geometry Tutor: An Intelligent Tutoring System for Proof-Writing with Construction. Proceedings of the Japan National Conference on Information and Systems in Education, (Vol. 125, pp. 443-450). 

Patton, M. Q. (2002). Qualitative research & evaluation methods. Sage.

Stylianou, D. A., Blanton, M. L., & Rotou, O. (2015). Undergraduate students’ understanding of proof: Relationships between proof conceptions, beliefs, and classroom experiences with learning proof. International Journal of Research in Undergraduate Mathematics Education, 1(1), 91–134.

Wang, K., & Su, Z. (2017). Interactive, intelligent tutoring for auxiliary constructions in geometry proofs. arXiv:1711.07154v1

Suggested Citation

Vestal, S., Buchbinder, O., and An, T. (2022, October). Using FullProof in Geometry for Teachers Courses. GeT: The News!, 4(1). https://www.gripumich.org/v4-i1-f2022/#using-fullproof-in-geometry-for-teachers-courses

A new working group: Engaging with the Student Learning Objectives (ESLO)

by Pat Herbst
University of Michigan

When we wrote the GeT Support proposal to NSF, we proposed to bring high school teachers of geometry into the conversations about Geometry courses for teachers. Because our initial moves toward forming the community showed that there already was a large diversity of perspectives among GeT instructors, we delayed that aspect of our proposed work. As the work of GeT: A Pencil has continued and fructified, we believe this might be a moment when we can bring in high school geometry teachers. Additionally, our community has, over time, involved other GeT Instructors who have not yet joined a working group. We are hoping to build an opportunity for that kind of participation.

There are two goals of this effort. The first goal is to promote a dialogue across high school geometry teachers and GeT instructors that can help make each group better aware of needs and goals. We surmise that GeT instructors could use additional knowledge about what the work of teaching high school geometry involves, and experienced high school geometry teachers could use more information about what happens in current GeT courses. Second, as our community has produced the first version of a set of essential student learning objectives for GeT courses and their elaborations–a living document, we think this new working group will provide a space to produce questions, reactions, and perhaps proposed revisions. Now that getapencil.org is up and running, the new working group might generate material to seed conversations in the forum. We also hope this group might propose some writing for the book in preparation. For example, it is reasonable to hope that some of the high school teachers in the group might collaborate to write about the SLOs from the high school teacher perspective–perhaps considering what they would like new hires to know and be able to do. It might also be reasonable to hope that GeT instructors in this new working group would have ideas for teaching or assessment activities that they would like to develop and connect with the SLOs through writing a book chapter.   

The new working group meets every other week on Thursdays at 4:00 pm ET.

Suggested Citation

Herbst P. (2022, October). A new working group: Engaging with the Student Learning Objectives (ESLO). GeT: The News!, 4(1). https://www.gripumich.org/v4-i1-f2022/#a-new-working-group-engaging-with-the-student-learning-objectives-eslo

Transformation Working Group Update

by Julia St. Goar
Merrimack College

Over the summer, the Transformation Working Group was facilitated by Steven Boyce and placed a special focus on a lesson study (Boyce et. al., 2021) involving Adinkra symbols originating from Ghana (Eglash et. al., n.d.). During this time, the group conducted research related to the lesson study and submitted work for publication. In the fall, the group will continue work on the lesson study, including working to make changes to the lesson itself. Currently, some members of the transformation group are conducting extra meetings outside of typical transformation group times to allow time to focus on this lesson.

This fall, the Transformation Working Group plans to spend a significant portion of its efforts on creating transformation geometry themed chapter proposals for The GeT course: Resources and Objectives for the Geometry Courses for Teachers. Once chapter proposals have been created, it is likely that the group meetings will be at least partially devoted to writing time for these chapters. Additionally, the group plans to discuss how we are preparing our future teachers to teach transformation geometry in schools, specifically how we are preparing them to choose appropriate tasks. Currently, theTransformation Working Group meets on Wednesdays at 11am (EDT) every other week.

References

Boyce, S., Ion, M., Lai, Y., McLeod, K., Pyzdrowski, L., Sears, R., & St. Goar, J. (2021, May 6). Best-Laid Co-Plans for a Lesson on Creating a Mathematical Definition. AMS Blogs: On Teaching and Learning Mathematics. https://blogs.ams.org/matheducation/2021/05/06/best-laid-co-plans-for-a-lesson-on-creating-a-mathematical-definition/ 

Eglash, R., Bennet, A., Krishnamoorthy, M., Sawyer, S., & Adali, S. (n.d.) Adinkra Background. Culturally Situated Design Tools. https://csdt.org/culture/adinkra/index.html 

Suggested Citation

St. Goar J. (2022, October). Transformations Working Group Update. GeT: The News!, 4(1). https://www.gripumich.org/v4-i1-f2022/#transformation-working-group-update

Teaching GeT working group update

by Nathaniel Miller
University of Northern Colorado

The Teaching GeT working group is very pleased to announce that the first public version of our Student Learning Outcomes (SLOs) for GeT courses is now completed and available at https://getapencil.org/student-learning-objectives/. Our plan is that these can still continue to evolve as they are more widely shared and as more voices enter the conversation about them. If you have thoughts or feedback about the SLOs, there is a forum on the website where they can be shared.

Members of the working group are now hard at work writing follow-up chapters about and around the SLOs to submit to the proposed book. Different subgroups are writing more about definitions; proofs; non-Euclidean geometry; the Adinkra lesson; technology; and possibly other topics. We welcome others to join us!

Suggested Citation

Miller N. (2022, October). Teaching GeT working group update. GeT: The News!, 4(1).
https://www.gripumich.org/v4-i1-f2022/#teaching-get-working-group-update

Get to know the community

Did you get promoted? Win a grant? Have a baby? Buy a house? We would love to feature your news, whether professional or personal! Email us at GRIP@umich.edu.

CALL FOR CHAPTER PROPOSALS: The GeT course: Resources and Objectives for the Geometry Courses for Teachers

by Dr. Amanda Brown
University of Michigan

Dr. Patricio Herbst
University of Michigan

Dr. Nathaniel Miller
University of Northern Colorado

Dr. Laura Pyzdrowski
West Virginia University 

Proposal Submission Deadline: November 28, 2022 
Anticipated Publication Time Frame: Summer 2024 

Most mathematics departments in universities that prepare secondary mathematics teachers offer a geometry class for teachers (GeT class, hereafter). However, there is a high degree of variability in the curriculum and instruction of these courses. In order to advance a shared understanding of what should be in a GeT course, a set of commonly-defined student learning outcomes (GeT SLOs) have been developed within an ongoing professional learning community called GeT: A Pencil—an open and growing community of about 40 plus GeT instructors gathered to work collectively in the pursuit of improving the geometric preparation of high school mathematics teachers. Recently, the Get: A Pencil community released a first public draft of the GeT SLOs and is currently soliciting feedback 

(https://getapencil.org/student-learning-objectives/). 

In order to publicize these SLOs, make them available to the larger mathematical community, and encourage further discussion about what a GeT course can and should be, we are soliciting chapter proposals for a forthcoming edited volume entitled The GeT course: Resources and Objectives for the Geometry Courses for Teachers. The edited volume will ultimately be considered for publication in the MAA Notes Series. The primary objective of this volume is to provide undergraduate instructors with ideas and resources to inform their design and development of GeT instruction, organized around the SLOs. 

We invite submissions on any topic relating to the GeT course and organized around the SLOs. Possible topics could include: 

• Reflections on, elaborations of, or reactions to one or more SLOs. 
• Examples of course designs and/or activities that address the SLOs in particularly novel or effective ways. 
• Ways to assess the SLOs. 
• Ideas for further work about the SLOs. 

We envision the volume serving as an outlet for instructors to share instructional and assessment resources that can be used to further illustrate and support the implementation of one or more of the SLOs within a GeT course. We also anticipate this volume will serve as an important outlet for individuals interested in sharing perspectives about the SLOs. Such perspectives can help shape future revisions of the SLOs as well as inform future collaborations around other undergraduate courses for teachers. Finally, we anticipate the volume serving to both elevate awareness amongst GeT instructors about the ongoing work, as well as identify the work ahead. Our hope is that, collectively, the contributions will help shape future revisions of the SLOs and contribute to the growing set of instructional artifacts that can help document the growing knowledge base for teaching the GeT course. 

Interested authors should submit an abstract of no more than 500 words in length by November 28, 2022. The abstract should provide a list of all authors and affiliations and an overview of the proposed chapter. If applicable, please identify the GeT SLOs that best align with the chapter. The abstract should be submitted as a Word document. The file name should match the proposed chapter title. To submit your abstract, please attach it to an email with “GeT Volume” in the subject line. 

Abstracts should be submitted to Amanda Brown (amilewsk@umich.edu) by November 28, 2022. Chapters will undergo peer review, with no guarantee of acceptance. 

Proposed timeline: 

November 20, 2022 Optional deadline for expressing interest in submitting a chapter proposal November 28, 2022 Outline/Abstract of proposed chapter (500 words) due January 25, 2023 Feedback and decision to prospective authors 

February 22, 2023 RUME pre-conference available for discussions and working teams 

For a more complete description of this volume, The GeT course: Resources and Objectives for the Geometry Courses for Teachers, as well as information about submission details and additional deadlines, go to: https://getapencil.org/get-writing-rfp/ 

Inquiries can be forwarded to any of the following:

Dr. Amanda M. Brown University of Michigan 734-615-1270 amilewsk@umich.edu	Dr. Patricio G. Herbst  University of Michigan pgherbst@umich.edu
Dr. Nathaniel Miller University of Northern Colorado nathaniel.miller@unco.edu	Dr. Laura Pyzdrowski West Virginia University lpyzdrow@math.wvu.edu

Upcoming Seminars

---

To submit a paper to be highlighted in a future newsletter, please fill out this form.